PLANETCALC Online calculators

Login
StudyMathGeometry

Calculation of elevation from horizontal distance and inclination angle with allowance for measurement error

This online calculator allows you to calculate the elevation from horizontal distance and inclination angle taking into account the mean square errors of measurements.

This page exists due to the efforts of the following people:

Timur

Timur

Created: 2023-12-02 17:40:20, Last updated: 2023-12-09 09:38:29
Creative Commons Attribution/Share-Alike License 3.0 (Unported)

This content is licensed under Creative Commons Attribution/Share-Alike License 3.0 (Unported). That means you may freely redistribute or modify this content under the same license conditions and must attribute the original author by placing a hyperlink from your site to this work https://planetcalc.com/9093/. Also, please do not modify any references to the original work (if any) contained in this content.

The calculation formulas are shown below the calculator

PLANETCALC, Calculation of elevation from horizontal distance and inclination angle with allowance for measurement error

Calculation of elevation from horizontal distance and inclination angle with allowance for measurement error

°
′
″
°
′
″
Digits after the decimal point: 2
Elevation, metres
 
MSE of elevation, metres
 

Calculation of elevation

In the figure below, the following notations are used:

  • S - line length
  • D - horizontal line projection, i.e. projection of the terrain line on a horizontal plane.
  • h - elevation, i.e. height difference of one point relative to another point
  • γ - angle of inclination of the line to the horizon

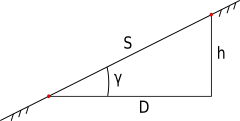
Horizontal distance
Horizontal distance



From fairly obvious geometric considerations, the elevation can be expressed in terms of horizontal distance and the angle of inclination to the horizon by the following formula:
h = D tg \gamma.

Mean square error of measurement

The accuracy of measurement is characterised by the mean square error of measurement (MSE). For quantities measured explicitly, the MSE is usually known. Elevation is measured indirectly, in this case, its value is calculated as a function of horizontal distance and inclination angle.

The general formula for the dependence of the MSE of the function calculation result on the MSE of its arguments is as follows:

If the quantity y is computed as a function of the arguments x_1, x_2, ..., x_n characterised by the MSE m_{x_1}, m_{x_1}, ..., m_{x_1}:
y = f(x_1, x_2, ..., x_n)

then the MSE of the calculated quantity y is equal to:
m_y=\sqrt{ \left(\frac{\delta f}{\delta x_1}\right)^2_0m^2_{x_1} + \left(\frac{\delta f}{\delta x_2}\right)^2_0m^2_{x_2} + ... + \left(\frac{\delta f}{\delta x_n}\right)^2_0m^2_{x_n} }

where \frac{\delta f}{\delta x_k} is the partial derivative of the function on the variable x_k. Index 0 means that the numerical value of the partial derivative obtained after substituting the numerical values of the arguments is taken.

In the case of the function h = D tg \gamma we have

m_h=\sqrt{ \left(\frac{\delta h}{\delta D}\right)^2_0m^2_{D} + \left(\frac{\delta h}{\delta \gamma}\right)^2_0m^2_{\gamma}} = \sqrt{ tg^2\gamma m^2_{D} + \left(\frac{D}{cos^2 \gamma}\right)^2 m^2_{\gamma}}

The inclination angle and the MSE of the inclination angle must be expressed in radians.

This is the formula used in the calculator to determine the MSE of the elevation.

URL copied to clipboard

Similar calculators

 elevation geodesy Geometry horizontal distance inclination inclination angle mean square error measurement
PLANETCALC, Calculation of elevation from horizontal distance and inclination angle with allowance for measurement error
Timur2023-12-09 09:38:29

Comments