Triangle values by coordinates of vertices
This online calculator calculates a set of triangle values: length of sides, angles, perimeter, and area by coordinates of its vertices
This page exists due to the efforts of the following people:
 | Timur
|
This content is licensed under Creative Commons Attribution/Share-Alike License 3.0 (Unported). That means you may freely redistribute or modify this content under the same license conditions and must attribute the original author by placing a hyperlink from your site to this work https://planetcalc.com/9423/. Also, please do not modify any references to the original work (if any) contained in this content.
This online calculator is designed to quickly calculate a number of characteristics of a triangle by the coordinates of its vertices. You enter the coordinates of the vertices A, B, and C. The calculator calculates the following values from the coordinates:
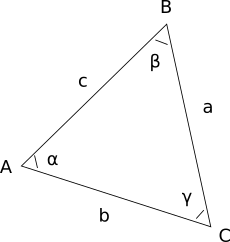
- the length of the side a - the side opposite to the vertex A
- the length of the side b - the side opposite to vertex B
- the length of the side c - the side opposite to vertex C
- the value of the angle α at the vertex A
- the value of the angle β at the vertex B
- the value of the angle γ at the vertex C
- the perimeter of the triangle
- area of a triangle
If you need something else, write in the comments, we'll add it. The formulas for calculating triangle values are described under the calculator.
Triangle values by coordinates of vertices
Vertex A
Vertex B
Vertex C
Calculating a triangle by the coordinates of the vertices
The lengths of the sides are found by the formula for calculating the distance between points in Cartesian coordinates
The angles are from the formulas for the dot product of vectors at the vertices.
The perimeter is found by simply adding the lengths of the sides.
The area of a triangle is found through the determinant
Comments